(content prepared by Yanne Chembo)
Edge-emitter semiconductor laser dynamics
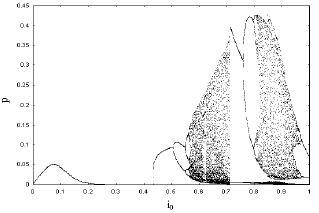Semiconductor lasers are intrinsically nonlinear components, and they display a very rich variety of complex behaviors. In particular, chaos may arise when the dynamical dimensionalty of the laser is increased. Today, several techniques are commonly used to induce chaos in semiconductor lasers, even though they can be gathered into two principal groups, namely parameter modulation and external feedback. Following the mainstream trend of research in chaos theory, great attention has been been paid to the collective dynamics of coupled semiconductor lasers in their chaotic regime. Along that line, the synchronization of such chaotic lasers became a focus of strong interest, and currently, the determination of the necessary and/or sufficient conditions for their synchronization is still a difficult challenge, which has turned to be crucial when chaotic semiconductor lasers became potentially eligible for hardware cryptography.
The figure displays the bifurcation diagram of a current-modulated semiconductor laser when the mean pumping current is increased (after ref. [1]). Such lasers have also been proven to display cluster synchronization [2]. On the other hand, we had also investigated the dynamics of external-cavity semiconductor lasers using the Lang-Kobayashi model, as well as their synchronization properties [3]. This research is led in collaboration with the University of Yaoundé I, Cameroon.
VCSELs dynamics
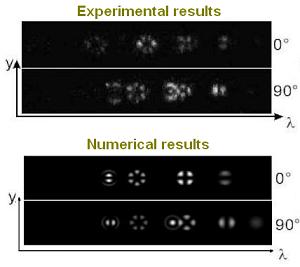Vertical-Cavity Surface Emitting Lasers (VCSELs) offer numerous advantages comparatively to their edge-emitter counterpart, To name just a few, VCSELs are intrinsically single-longitudinal mode lasers, and they have a significantly lower threshold current, as well as a lower power consumption. They are very cost effective because they can simultaneously be fabricated in a planar structure, and then tested "on wafer"; this planar structure also allows for easy integration in two-dimensional arrays. The circular cross-section of VCSELs produces lowdivergence beams (thus limiting the need of corrective optics), and enables a highly efficient laser-fiber coupling. VCSELs are nowadays particularly spread in optical fiber data transmission (mostly in gigabit-ethernet networks), free-space optical communications, absorption spectroscopy, laser printers, sensors, pointers and trackers.
A difficult challenge in most of VCSELs applications is the design high-power, single-mode, and single-polarization output beams. This is for example a critical issue in optical communication networks with ultra-dense wavelength-division multiplexing (UD-WDM), where the spectral spacing between adjacent channels can be as low as 25 GHz. The control of the emission properties of VCSELs can be achieved using polarization- and frequency-selective feedback. The figure (after ref. [4]) shows how the numerical simulations based on a modal expansion model enable to recover satisfyingly the main features of the emitted radiation: polarization, wavelength, and spatial orientation. The experimental set-up has been developed at the Darmstadt University of Techology, Germany.
Optoelectronic systems
Optoelectronic oscillators are becoming increasingly important hybrid systems in communication technology. They typically consist in a semiconductor laser feeding a Mach-Zehnder modulator, whose output is delayed in a fiber delay line, detected with a photo-detector, amplified, filtered and finally fed back to the radio-frequency input of the modulator. Typically, this same architecture can be declined into two different technologies, depending on the bandwidth of the filter and the length of the fiber delay line inserted into path of the feedback loop. When the filter bandwidth is large and the fiber delay line is short, the system can display wideband hyperchaos; on the other hand, when the filter is narrowband and the delay line is long, the system outputs a single-mode signal. Other configurations are also possible, thereby enabling curious dynamical features such as narrowband hyperchaos.
The figure (after ref. [5]) displays the experimental set up of an optoelecronic pulse generator, that can be modelized with an hybrid set of equations, namely a delay-differential equation and a nonlinear Schrödinger equation. Activities in this area include the nonlinear and stochastic dynamics of wideband ([6], [7]) and and narrowband optoelectronic oscillators ([8], [9], [10]).