(content prepared by Yanne Chembo)
Delayed dynamical systems
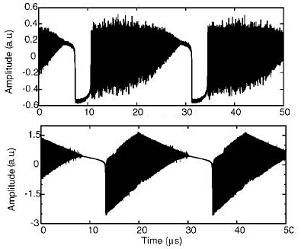 Delayed dynamical systems are ubiquitous. Typically, in these systems, the dynamical state at a given time depends on the state of the system at an anterior time, and the delay time generally coincides with the propagation time of the fed back signal. In fact, delayed variables should be taken into account in absolutely all dynamical systems, because the propagation times of the various interactions are never null. But as long as they are small comparatively to the relevant timescales of the system's dynamics, these delays can be neglected and the dynamical equations degenerate into a flow of Ordinary Differential Equations (ODE), which inherently assume instantaneous interactions. Sometimes however, the delay time has the same order of magnitude as the system's typical timescales. This may for example occur in ultrafast systems, of for particularly long delay times. In these cases, the delay can not be neglected anymore and the system should rather be modelized with a Delay Differential Equation (DDE). Even though it is not apparent at first view, DDEs are very complex equations: at the opposite of ODEs, they are infinite-dimensional, because their initial condition is a continuous and compact interval. Therefore, the simple fact to introduce and account for a delay time in a dynamical system increases its dimensionality to infinity, and thereby opens the way to a wide variety of very complex behaviors.
Delayed dynamical systems are ubiquitous. Typically, in these systems, the dynamical state at a given time depends on the state of the system at an anterior time, and the delay time generally coincides with the propagation time of the fed back signal. In fact, delayed variables should be taken into account in absolutely all dynamical systems, because the propagation times of the various interactions are never null. But as long as they are small comparatively to the relevant timescales of the system's dynamics, these delays can be neglected and the dynamical equations degenerate into a flow of Ordinary Differential Equations (ODE), which inherently assume instantaneous interactions. Sometimes however, the delay time has the same order of magnitude as the system's typical timescales. This may for example occur in ultrafast systems, of for particularly long delay times. In these cases, the delay can not be neglected anymore and the system should rather be modelized with a Delay Differential Equation (DDE). Even though it is not apparent at first view, DDEs are very complex equations: at the opposite of ODEs, they are infinite-dimensional, because their initial condition is a continuous and compact interval. Therefore, the simple fact to introduce and account for a delay time in a dynamical system increases its dimensionality to infinity, and thereby opens the way to a wide variety of very complex behaviors.
The figure (after ref. [1]) displays the experimental (top) and numerical (bottom) timetraces of the so-called chaotic breathers, that can emerge in the wideband configuration depending on the parameters. Another work on complex behavior in wideband optoelectronic oscillators is ref. [2]. On the other hand, we have also developed a sustained research activity to investigate the nonlinear dynamics of external-cavity semiconductor lasers [3], [4], and of narrow-band microwave optoelectronic oscillators ([5], [6], [7]).
Nonlinear oscillations, chaos control and synchronization
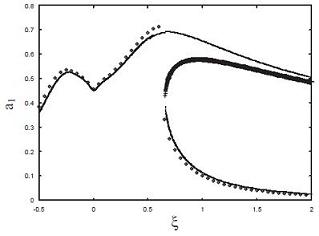 Fundamental problems of nonlinear dynamics are still the focus of intense research activities worldwide. In particular, the study of nonlinear and chaotic oscillations naturally find applications in a wide variety of scientific and technological poblems. As far as coupled nonlinear systems are concerned, it was thought for a long time that the natural extension of chaos in spatially coupled oscillators is spatiotemporal chaos, that is, a state of simultaneous chaos in the spatial and temporal domains. However, phenomena of self-organization and spatial coherence have effectively been observed in coupled chaotic oscillators, and have triggered off a tremendous interest for the study of their spatiotemporal dynamics. Applications rapidly appeared to be wide: pattern formation modeling, coherent collective behavior in nonlinear physical, biological and ecological systems, oscillatory chemical processes, extended neuronal networks, etc. For a number of oscillators restricted to two, emphasis is commonly laid upon the state of synchronization, which occurs when the two identical oscillators continuously remain in step with each other.
Fundamental problems of nonlinear dynamics are still the focus of intense research activities worldwide. In particular, the study of nonlinear and chaotic oscillations naturally find applications in a wide variety of scientific and technological poblems. As far as coupled nonlinear systems are concerned, it was thought for a long time that the natural extension of chaos in spatially coupled oscillators is spatiotemporal chaos, that is, a state of simultaneous chaos in the spatial and temporal domains. However, phenomena of self-organization and spatial coherence have effectively been observed in coupled chaotic oscillators, and have triggered off a tremendous interest for the study of their spatiotemporal dynamics. Applications rapidly appeared to be wide: pattern formation modeling, coherent collective behavior in nonlinear physical, biological and ecological systems, oscillatory chemical processes, extended neuronal networks, etc. For a number of oscillators restricted to two, emphasis is commonly laid upon the state of synchronization, which occurs when the two identical oscillators continuously remain in step with each other.
The figure displays the hysteritic behavior of an electrostatic transducer (after ref. [8]). The lines represent the analytical solutions (thin for stable nonlinear oscillations, thick for unstable), while the symbols stand for the numerical simulations. Other early works on this topic include refs. [9], [10], [11], [12], and [13], undertaken at the University of Yaoundé I.

